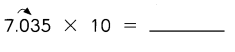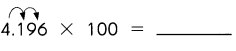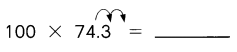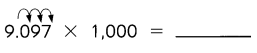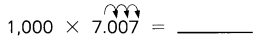Practice the problems of Math in Focus Grade 5 Workbook Answer Key Chapter 9 Practice 2 Multiplying by Tens, Hundreds, and Thousands to score better marks in the exam.
Math in Focus Grade 5 Chapter 9 Practice 2 Answer Key Multiplying by Tens, Hundreds, and Thousands
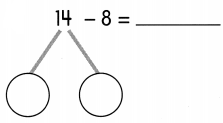
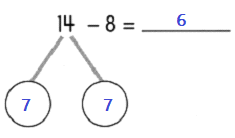
Complete. Draw chips and use arrows to show how the chips move. Then fill in the blanks.
Question 1.
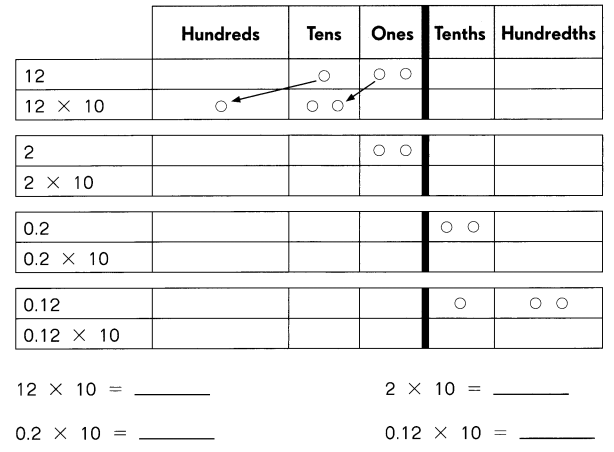
Answer:
12 x 10 = 120
2 x 10 = 20
0.2 x 10 = 2
0.12 x 10 = 1.2
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Multiply.
Question 2.
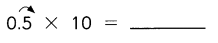
Answer: 5
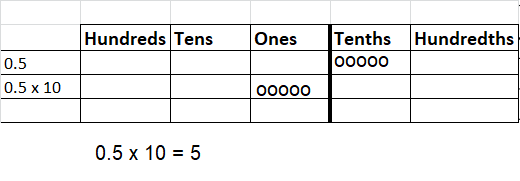
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 3.
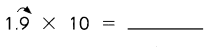
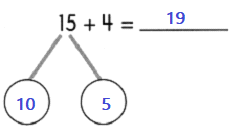
Answer: 19
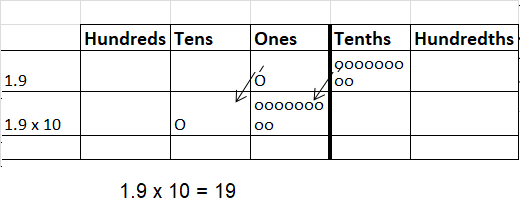
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 4.
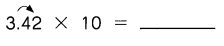
Answer: 34.2
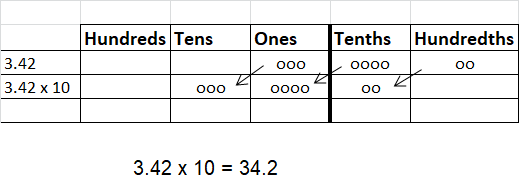
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 5.
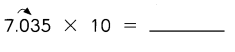
Answer: 70.35
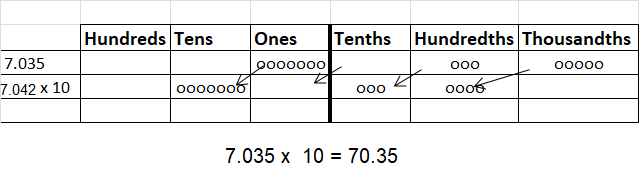
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 6.
10 × 7.9 = ___
Answer:79
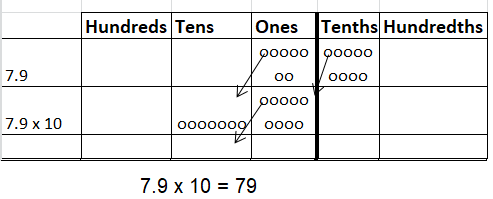
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 7.
10 × 4.8 = ___
Answer: 48
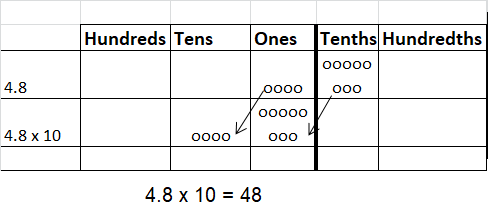
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 8.
10 × 27.54 = ___
Answer: 275.4
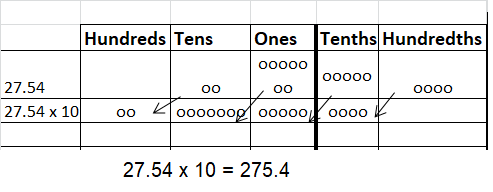
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 9.
10 × 12.009 = ____
Answer: 120.09
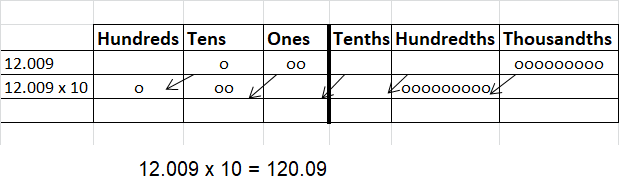
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 10.
0.7 × ___ = 7
Answer: 10
0.7 x 10 = 7
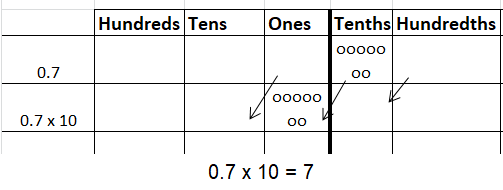
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 11.
15.72 × ___ = 157.2
Answer: 10
15.72 x 10 = 157.2
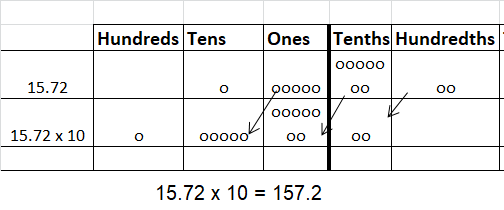
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 12.
10 × ___ = 534.2
Answer: 534.2
10 x 53.42
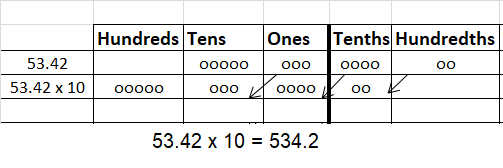
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 13.
___ × 10 = 19.07
Answer: 1.907
1.907 x 10 = 190.7
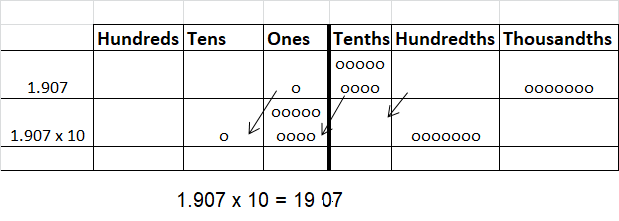
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Complete
Example
8 × 50 = (8 × 5) × 10
= 40 × 10
= 400
So, 8 × 50 = 400
Question 14.
0.8 × 50 = (0.8 × 5) × ___
= ___ × 10
= ____
So, 0.8 × 50 = ___
Answer:
0.8 × 50 = (0.8 × 5) × 10
= 4.0 × 10
= 40
So, 0.8 × 50 = 40
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 15.
0.88 × 50 = (0.88 × ___) × 10
= ___ × 10
= ____
So, 0.88 × 50 = ___
Answer:
0.88 × 50 = (0.88 × 5) × 10
= 4.4 × 10
= 44
So, 0.88 × 50 = 44
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Find each product.
Question 16.
0.9 × 40 = ____
Answer:
0.9 × 40 = (0.9 × 4) × 10
= 3.6 × 10
= 36
So, 0.9 × 40 = 36
Explanation:
Separate 40 as 4 ones and 1 tenths,
then multiply with ones first and later tens.
Question 17.
1.5 × 60 = ___
Answer:
1.5 × 60 = (1.5 × 6) × 10
= 9.0 × 10
= 90
So, 1.5 × 60 = 90
Explanation:
Separate 60 as 6 ones and 1 tenths,
then multiply with ones first and later tens.
Question 18.
0.05 × 80 = ____
Answer:
0.05 × 80 = (0.05 × 8) × 10
= 0.4 × 10
= 4
So, 0.05 × 80 = 4
Explanation:
Separate 80 as 8 ones and 1 tenths,
then multiply with ones first and later tens.
Question 19.
9.17 × 70 = ___
Answer:
9.17 x 70 = (9.17 x 7) x 10
=64.19 x 10
=641.9
So, 9.17 x 70 = 641.9
Explanation:
Separate 70 as 7 ones and 1 tenths,
then multiply with ones first and later tens.
Question 20.
6.358 × 30 = ___
Answer:
6.358 x 30 = (6.358 x 3) x 10
=19.074 x 10
=190.74
So,6.358x 30 = 190.74
Explanation:
Separate 30 as 3 ones and 1 tenths,
then multiply with ones first and later tens.
Question 21.
34.6 × 50 = ____
Answer:
34.6 x 50 = (34.6 x 5) x 10
= 173 x 10
= 1730
So, 34.6 x 50 =1730
Explanation:
Separate 50 as 5 ones and 1 tenths,
then multiply with ones first and later tens.
Question 22.
41.32 × 60 = ___
Answer:
41.32 x 60 = (41.32 x 6) x 10
= 247.92 x 10
= 2479.2
So, 41.32 x 60= 2479.2
Explanation:
Separate 60 as 6 ones and 1 tenths,
then multiply with ones first and later tens.
Question 23.
23.05 × 40 = ___
Answer:
23.05 x 40 = (23.05 x 4) x 10
=92.2 x 10
=922
So, 23.05 x 40 = 92.2
Explanation:
Separate 40 as 4 ones and 1 tenths,
then multiply with ones first and later tens.
Question 24.
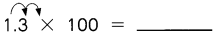
Answer: 130
Explanation:
1.3 x 100 = (1.3 x10) x 10
=13 x 10
=130
So, 1.3 x 100 = 130
Question 25.
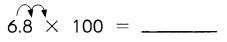
Answer: 680
Explanation:
6.8 x 100 = (6.8 x 10) x 10
= 68 x 10
= 680
So, 6.8 x 100 = 680
Question 26.
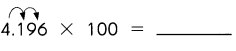
Answer: 419.6
Explanation:
4.196 x 100 = (4.196 x 10) x 10
= 41.96 x 10
= 419.6
So, 4.196 x 100 = 419.6
Question 27.
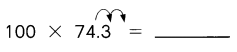
Answer: 7430
Explanation:
74.3 x 100 = (74.3 x 10) x 10
= 743 x 10
= 7430
So, 74.3 x 100 = 7430
Question 28.
46.8 × 1oo = ______
Answer: 4680
Explanation:
46.8 x 100 = (46.8 x 10) x 10
=468 x 10
= 4680
Question 29.
4.68 × 100 = ___
Answer: 468
Explanation:
4.68 x 100 = (4.68 x 10) x 10
=46.8 x 10
= 468
So, 4.68 x 100 = 468
Question 30.
5.095 × 100 = ______
Answer: 509.5
Explanation:
5.095 x 100 = (5.095 x 10 )x 10
= 50.95 x 10
= 509.5
So, 5.095 x 100 = 509.5
Question 31.
100 × 50.95 = ____
Answer: 5095
Explanation:
50.95 x 100 = (50.95 x 10) x 10
= 509.5 x 10
= 5095
So, 50.95 x 100 = 5095
Multiply.
Question 32.
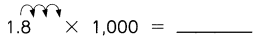
Answer: 1800
Explanation:
1.8 x 1000 = (1.8 x 10) x 10 x 10
= 18 x 10 x 10
=180 x 10
=1800
So, 1.8 x 1000 = 1800
Question 33.
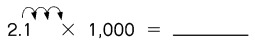
Answer: 2100
Explanation:
2.1 x 1000 = (2.1 x 10) x 10 x 10
=21 x 10 x 10
= 210 x 10
= 2100
So, 2.1 x 1000 = 2100
Question 34.
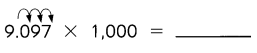
Answer: 9097
Explanation:
9.097 x 1000 = (9.097 x 10) x 10 x 10
=90.97 x 10 x 10
= 909.7 x 10
=9097
So, 9.097 x 1000 = 9097
Question 35.
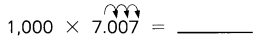
Answer: 7007
Explanation:
7.007 x 1000 = (7.007 x 10) x 10 x 10
= 70.07 x 10 x 10
= 700.7 x 10
= 7007
So, 7.007 x 1000 = 7007
Question 36.
2.74 × 1,000 = ______
Answer: 2740
Explanation:
2.74 x 1000= (2.74 x 10) x 10 x 10
=27.4 x 10 x 10
=274 x 10
= 2740
Question 37.
27.4 × 1,000 = ____
Answer: 27400
Explanation:
27.4 x 1000 = (27.4 x 10) x 10 x 10
=274 x 10 x10
=2740 x 10
27400
Question 38.
1,000 × 10.81 = ______
Answer: 10810
Explanation:
10.81 x 1000 =(10.81 x 10) x 10 x 10
= 108.1 x10 x 10
= 1081 x 10
= 10810
So, 10.81 x 1000 = 10810
Question 39.
108.1 × 1,000 = ____
Answer: 108100
Explanation:
108.1 x1000 =(108.1 x 10) x 10 x 10
= 1081 x 10 x 10
= 10810 x 10
= 108100
So, 108.1 x 1000 = 108100
Complete.
Example
1.2 = 0.12 × 10
= 0.012 × 100
Question 40.
360 = 36 × ____
= 3.6 × ____
= 0.36 × _____
Answer:
360 = 36 × 10
= 3.6 × 100
= 0.36 × 1000
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 41.
438 = __ × 10
= ___ × 100
= ___ × 1,000
Answer:
438 = 43.8 × 10
= 4.38 × 100
=0.438 × 1,000
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Question 42.
7,256 = ______ × 10
= ____ × 100
= ____ × 1,000
Answer:
7,256 = 725.6 × 10
= 72.56 × 100
= 7.256 × 1,000
Explanation:
To multiply decimals, first multiply as if there is no decimal.
Next, count the number of digits after the decimal in each factor.
Finally, put the same number of digits behind the decimal in the product.
Multiply.
Example
0.3 × 700 = (0.3 × 7) × 1oo
= 2.1 × 1oo = 210
So, 0.3 × 700 = 210
Question 43.
0.003 × 700 = (0.003 × __) × 100
= ____ × 1oo = ____
So, 0.003 × 700 = ___.
Answer:
0.003 × 700 = (0.003 × 7) × 100
= 0.021 × 1oo = 2.1
So, 0.003 × 700 = 2.1.
Explanation:
The place value of a number is the value represented by a digit in a number based on its position in the number.
While a place value is the value a digit holds to be at the place in the number, on the other hand.
First separate the hundredth place value,
and multiply the given numbers with the number .
Question 44.
0.03 × 2,000 = (0.03 × __) × 1,000
= ______ × 1,000 = ______
So, 0.03 × 2,000 = ____.
Answer:
0.03 × 2,000 = (0.03 × 2) × 1,000
= 0.06 × 1,000 = 60
So, 0.03 × 2,000 = 60.
Explanation:
The place value of a number is the value represented by a digit in a number based on its position in the number.
While a place value is the value a digit holds to be at the place in the number, on the other hand.
First separate the place value,
and multiply the given numbers with the number .
Question 45.
0.003 × 2,000 = (0.003 × __________) × 1,000
= __________× 1,000 = _______.
So, 0.003 × 2000 = __________.
Answer:
0.003 × 2,000 = (0.003 × 2) × 1,000
= 0.006× 1,000 = 6
So, 0.003 × 2000 = 6.
Explanation:
The place value of a number is the value represented by a digit in a number based on its position in the number.
While a place value is the value a digit holds to be at the place in the number, on the other hand.
First separate the place value,
and multiply the given numbers with the number .
Find each product.
Question 46.
4.5 × 200 = _______
Answer: 900
Explanation:
(4.5 x 2) x 10 x 10
= 9 x 10 x 10
= 90 x 10
= 900
So, 4.5 x 200 = 900
Question 47.
0.49 × 300 = ___
Answer: 147
Explanation:
0.49 x 300 = (0.49 x 3) x 10 x 10
= 1.47 x 10 x 10
= 14.7 x 10
= 147
So, 0.49 x 300 = 147
Question 48.
3.148 × 500 = ______
Answer: 1,574
Explanation:
3.148 x 500 = (3.148 x 5) x 10 x 10
= 15.74 x 10 x 10
= 157.4 x 10
= 1574
So, 3.147 x 500 = 1574
Question 49.
2.27 × 700 = ___
Answer: 1,589
Explanation:
2.27 x 700 = (2.27 x 7) x 10 x 10
= 15.89 x10 x10
= 158.9 x 10
= 1589
So , 2.27 x 700 = 1589
Question 50.
900 × 3.18 ______
Answer: 2,862
Explanation:
3.18 x 900 = (3.18 x 9) x 10 x 10
= 28.62 x 10 x 10
= 286.2 x 10
= 2862
So, 3.18 x 900 = 2862
Question 51.
1.8 × 2,000 = ___
Answer: 36,00
Explanation:
1.8 x 2000 = (1.8 x 20) x 10 x 10
= 36 x 10 x 10
=360 x 10
=3600
So, 1.8 x 2000 = 3600
Question 52.
4,000 × 2.5 = _______
Answer: 10,000
Explanation:
2.5 x 4000 = (2.5 x 40) x 10 x 10
= 100 x10 x10
= 1000 x 10
= 10000
So, 2.5 x 4000 = 10000
Question 53.
72.5 × 6,000 = ___
Answer: 435,000
Explanation:
72.5 x 6000 = ( 72.5 x 60) x 10 x 10
= 4350 x10 x10
= 43500 x 10
= 435000
So, 72.5 x 6000 = 435000
Question 54.
1.75 × 8,000 = ______
Answer: 14,000
Explanation:
1.75 x 8000 = (1.75 x 80) x 10 x 10
= 140 x10 x10
= 1400 x10
= 14000
So 1.75 x 8000 = 14000
Question 55.
4.19 × 9,000 = ___
Answer: 37,710
Explanation:
4.19 x 9000 = (4.19 x90) x 10 x 10
= 377.1 x10 x10
= 3771 x 10
= 37710
So, 4.19 x 9000 = 37710
![]() 6 = 4
6 = 4![]() 5 = 12
5 = 12![]() 9 = 7
9 = 7![]() 7 = 16
7 = 16![]() 3 = 14
3 = 14![]() 6 = 20
6 = 20![]() 2 = 15
2 = 15![]() 8 = 20
8 = 20
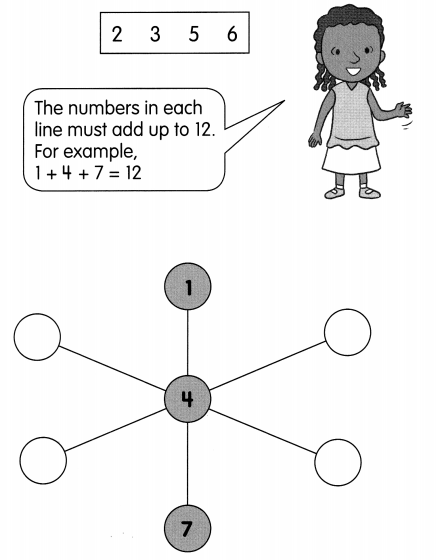
![]() with any of these numbers. Use each number once.
with any of these numbers. Use each number once.