Math in Focus Grade 7 Chapter 8 Lesson 8.4 Answer Key Finding Volume and Surface Area of Spheres

This handy Math in Focus Grade 7 Workbook Answer Key Chapter 8 Lesson 8.4 Finding Volume and Surface Area of Spheres detailed solutions for the textbook questions.

Math in Focus Grade 7 Course 2 B Chapter 8 Lesson 8.4 Answer Key Finding Volume and Surface Area of Spheres

Math in Focus Grade 7 Chapter 8 Lesson 8.4 Guided Practice Answer Key

Solve.

Question 1.
The diameter of a sphere is 8.8 meters. What is the volume of the sphere? Round your answer to the nearest hundredth. Use 3.14 as an approximation for π.

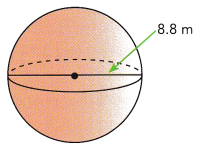
Radius of the sphere:
8.8 ÷ = m The radius is half the diameter.
Volume of the sphere:
\(\frac{4}{3}\)πr³ ≈ \(\frac{4}{3}\) ∙ ∙ ∙ ∙ Use the formula for the volume of a sphere.
= Multiply.
≈ m³ Round to the nearest hundredth.
The volume of the sphere is about cubic meters.
Answer: The volume of the sphere is about 356.63 cubic meters.

Explanation:
Given, diameter of a sphere is 8.8 meters.
Then r = 4.4 m
We know that the volume of the sphere is V =  \(\frac{4}{3}\)πr³
V = \(\frac{4}{3}\) × 3.14 × 4.4 × 4.4 × 4.4
V = \(\frac{1069.9}{3}\)
V = 356.63 m³
So, The volume of the sphere is about 356.63 cubic meters.

Question 2.
Diane bought a spherical ball made of quartz at a garage sale. The volume of the ball is 1,450 cubic centimeters. What is the radius of the ball to the nearest centimeter? Use 3.14 as an approximation for π.

The radius of the spherical ball is about centimeters.
Answer: The radius of the sphere is  7 meters.

Explanation:
Given, The volume of the sphere is about 1450 cubic meters.
Then r = ?
We know that the volume of the sphere is V =  \(\frac{4}{3}\)πr³
1450 = \(\frac{4}{3}\) × 3.14 ×r³
r³ = \(\frac{1450 × 3}{3.14 × 4}\)
r³ = \(\frac{4350}{12.56}\)
r³= 346.33
r = 7
So, The radius of the sphere is  7 meters.

Hands-On Activity

Materials:

• string
• hemisphere
• cylinder that has the same radius and height twice the radius of the hemisphere

Work in pairs.

STEP 1: Wrap the string tightly around the hemisphere as shown in the diagram. Measure the length of the string used, and then use the same length of string to wrap around the curved surface of the cylinder.

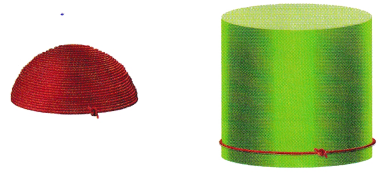
STEP 2: How much of the curved surface of the cylinder does the string cover? If you wrap the whole sphere with string, how much of the curved surface of the cylinder would the same string cover?

STEP 3: How is the surface area of the sphere related to the area of the curved surface of the cylinder?

STEP 4: Copy and complete the following to write a formula for the surface area of a sphere. (Hint: The height of a cylinder is twice the radius of a sphere.)
Surface area of a sphere = Area of cylinder’s curved surface

Solve.

Question 3.
What is the surface area of a sphere with a radius of 6 centimeters? Use 3.14 as an approximation for π.
Answer: The surface area of a sphere is  452.16 cm²

Explanation:
Given, r = 6 cm,
The surface area of a sphere is A = 4πr²
= 4 × 3.14 × 6 × 6
= 452.16 cm²
So, The surface area of a sphere is  452.16 cm²

Question 4.
A spherical rubber ball has a surface area of 3,215.36 square centimeters. What is the radius of the rubber ball to the nearest centimeter? Use 3.14 as an approximation for π.

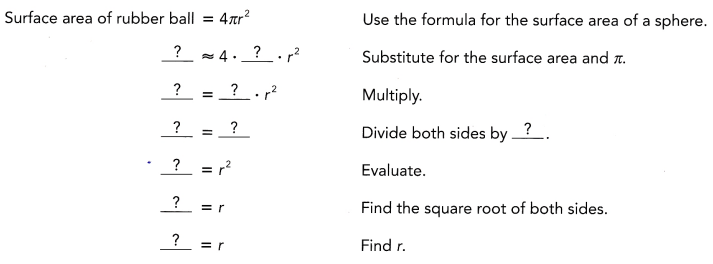
The radius of the rubber ball is about centimeters.
Answer: The radius of a sphere is 16 cm.

Explanation:
rubber ball has a surface area of 3,215.36 square centimeters.
Given, A = 3,215.36 cm²,
The surface area of a sphere is A = 4πr²
3,215.36 = 4 × 3.14 × r²
r² = \(\frac{3,215.36}{3.14 × 4}\)
r² = 256
r = 16
So, The radius of a sphere is 16 cm.

Math in Focus Course 2B Practice 8.4 Answer Key

For this practice, you may use a calculator and use 3.14 as an approximation for π. Round your answers to the nearest tenth when you can.

Solve.

Question 1.
What is the volume of a sphere with a radius of 5 centimeters?
Answer: The volume of the sphere is about 523.33 cm³.

Explanation:
Given,  r = 5 cm
We know that the volume of the sphere is V =  \(\frac{4}{3}\)πr³
V = \(\frac{4}{3}\) × 3.14 × 5 × 5 × 5
V = \(\frac{1570}{3}\)
V = 523.33 cm³
So, The volume of the sphere is about 523.33 cm³.

Question 2.
What is the surface area of a sphere with a radius of 4.4 centimeters?
Answer: The surface area of a sphere is  243.16 cm²

Explanation:
Given, r = 4.4 cm,
The surface area of a sphere is A = 4πr²
= 4 × 3.14 × 4.4 × 4.4
= 243.16 cm²
So, The surface area of a sphere is  243.16 cm²

Question 3.
A fully inflated beach ball has a radius of 10 inches.
a) What is the surface area of the beach ball?
Answer: The surface area of a sphere is  1256 in²

Explanation:
Given, r = 10 in,
The surface area of a sphere is A = 4πr²
= 4 × 3.14 ×10 × 10
= 1256 in²
So, The surface area of a sphere is  1256 in²

b) What is the volume of air inside the beach ball?
Answer:  The volume of the air inside the ball is about 4186.6 in³.

Explanation:
Given,  r = 10 in
We know that the volume of the sphere is V =  \(\frac{4}{3}\)πr³
V = \(\frac{4}{3}\) × 3.14 × 10 × 10 × 10
V = 4186.6 in³
So, The volume of the air inside the ball is about 4186.6 in³.

Question 4.
What is the surface area of a sphere.
a) with a diameter of 28.6 centimeters?
Answer: The surface area of a sphere is  2,568 cm²

Explanation:
Given, d = 28.6
Then, r = 14.3 cm,
The surface area of a sphere is A = 4πr²
= 4 × 3.14 × 14.3 × 14.3
= 2,568 cm²
So, The surface area of a sphere is  2,568 cm²

b) with a volume of 3,680 cubic centimeters?
Answer:  The surface area of a sphere is  1133.54 cm²

Explanation:
Given, The volume of the sphere is about 3680 cubic meters.
Then r = ?
We know that the volume of the sphere is V =  \(\frac{4}{3}\)πr³
3680 = \(\frac{4}{3}\) × 3.14 ×r³
r³ = \(\frac{3680× 3}{3.14 × 4}\)
r³ = \(\frac{11040}{12.56}\)
r³= 878.9
r = 9.5
So, The radius of the sphere is  9.5 cm.

Then, r = 9.5 cm,
The surface area of a sphere is A = 4πr²
= 4 × 3.14 × 9.5 × 9.5
= 1133.54 cm²
So, The surface area of a sphere is  1133.54 cm²

Question 5.
A billiard ball has a surface area of 84 square centimeters. What is the radius of the billiard ball?
Answer: The radius of a billiard ball is 2..5 cm.v

Explanation:
billiard ball has a surface area of 84 square centimeters.
Given, A = 84 cm²,
The surface area of a sphere is A = 4πr²
84 = 4 × 3.14 × r²
r² = \(\frac{84}{3.14 × 4}\)
r² = 6.68
r = 2.5
So, The radius of a billiard ball is 2..5 cm.

Question 6.
A bowl is in the shape of a hemisphere. The radius of the bowl is 10 centimeters. How many liters of water can the bowl hold? (1,000 cm³ = 1 liter)

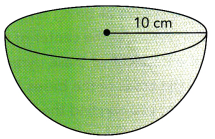
Answer: the bowl can hold 0.020 liters of water

Explanation:
Volume of the hemisphere  V = \(\frac{2}{3}\)πr
Given, r = 10 cm
V = \(\frac{2}{3}\) × 3.14 × 10
V = 20.9 cm³
The volume of the hemisphere is 20.9 cm³

Then, Given that 1000 cm = 1 liter, and
we want to know how many liters the bowl can hold.
= \(\frac{20.93}{1000}\)
= 0.020 liters
So, the bowl can hold 0.020 liters of water

Question 7.
Math Journal
a) What dimension of a sphere do you need to find its surface area and volume?
Answer: We need the dimension of radius of a sphere to find the surface area and its volume

b) Suppose a sphere has a radius greater than 1 unit. If you double the radius, which value will increase by a greater amount, the volume or the surface area of the sphere? Explain your thinking.
Answer: The surface area increases. with the square of the radius.
If you double the radius you quadruple the surface area

Explanation:
If r is the radius of a sphere its surface area = 4πr².
If the radius is doubled then its surface area = 4π(2r)² = 4 × 4πr².
So the surface area becomes 4-fold or the increase in surface area is 300%.

Question 8.
A basketball is shipped in a cube-shaped box. The basketball just touches the sides of the box, as shown.

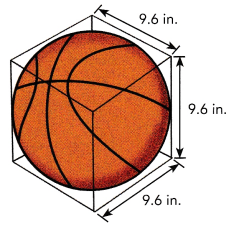
a) What is the radius of the basketball?
Answer: the radius of the basketball is  4.8 in.

Explanation:
Given, diameter of a sphere is 9.6 in.
r = \(\frac{d}{2}\)
= \(\frac{9.6}{2}\)
= 4.8 in.
Then r = 4.8 in.
So, the radius of the basketball is  4.8 in.

b) What is the volume of the basketball?
Answer: The volume of the basketball is about 463 in³.

Explanation:
Given,  r = 4.8 in
We know that the volume of the sphere is V =  \(\frac{4}{3}\)πr³
V = \(\frac{4}{3}\) × 3.14 × 4.8 × 4.8 × 4.8
V = \(\frac{1389}{3}\)
V = 463 in³
So, The volume of the basketball is about 463 in³.

c) About what percent of the space in the cube is occupied by the basketball?
Answer: 52.3 % of the cube is occupied by the baseball

Explanation:
Given, the cube side a = 9.6 in
The volume of a cube  V = a³
V = 9.6 × 9.6 × 9.6
V = 884.73 in³
The volume of a cube is 884.73 in³

The volume of the basketball is about 463 in³.
So, around 52.3 % of the cube is occupied by the baseball

Question 9.
A solid metal ball with a radius of 10 inches is melted and made into smaller spherical metal balls with a radius of 2 inches each. How many smaller spherical balls can be made?
Answer: total of 125 smaller spherical metal balls can be made

Explanation:
r = 10 in
Volume of the metal ball  V =  \(\frac{4}{3}\)πr³
V = \(\frac{4}{3}\) × 3.14 × 10 × 10 × 10
V = 4186.6 in³
So, The volume of the sphere is about 4186.6 in³

Volume of the smaller spherical metal balls , with r = 2 in.
V =  \(\frac{4}{3}\)πr³
V = \(\frac{4}{3}\) × 3.14 × 2 × 2 × 2
V = 33.49 in³

So, divide the volumes, we get
= \(\frac{4186.6}{33.49}\)
= 125
Hence,  total of 125 smaller spherical metal balls can be made

Question 10.
Nathan cuts a clay sphere in half to get two hemispheres. He measures the circumference of the hemispheres to be 175.84 centimeters.

a) What is the radius of each hemisphere?
Answer: The radius of each hemisphere is 28 cm

Explanation:
Given, C = 175.84 cm
The circumference of the hemisphere is C = 2πr
175.84 = 2 × 3.14 × r
r = \(\frac{175.84}{6.28}\)
r = 28 cm
So, The radius of each hemisphere is 28 cm

b) What is the total surface area of each solid hemisphere?
Answer: The  total surface area of each hemisphere is  7,385.28 cm²

Explanation:
the total surface area of each hemisphere is A = 3πr²
= 3 × 3.14 × 28 × 28
= 7,385.28 cm²
So, The  total surface area of each hemisphere is  7,385.28 cm²

Question 11.
The volume of a sphere is 3,052.08 cubic meters.
a) What is the radius of the sphere?
Answer: The radius of the sphere is  9 cm.

Explanation:
Given, The volume of the sphere is about 3,052.08 cubic meters.
Then r = ?
We know that the volume of the sphere is V =  \(\frac{4}{3}\)πr³
3,052.08 = \(\frac{4}{3}\) × 3.14 ×r³
r³ = \(\frac{3,052.08× 3}{3.14 × 4}\)
r³ = \(\frac{91529}{12.56}\)
r³= 729
r = 9
So, The radius of the sphere is  9 cm.

b) What is the surface area of the sphere?
Answer: The surface area of a sphere is  1017.36 cm²

Explanation:
Then, r = 9 cm,
The surface area of a sphere is A = 4πr²
= 4 × 3.14 × 9 × 9
= 1017.36 cm²
So, The surface area of a sphere is  1017.36 cm²

Question 12.
Once you know how to find the surface area of a sphere, you can use the surface area formula to see why the volume formula works.
Think of a sphere as being divided up into hundreds of “pyramids” that have a common vertex at the center of the sphere. The surface of the sphere is made up of the bases of all these pyramids. The height of each pyramid is r, and you can call the areas of the bases B₁, B₂, B₃, and so on. To find the surface area of the sphere, you can find the sum of the areas of all the bases.

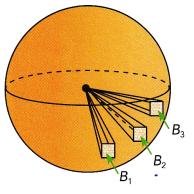
Show why the volume formula works by supplying a reason for each step below.

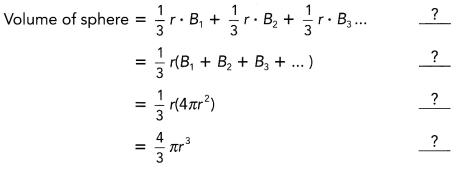
Answer:
Add the volumes of the pyramids to get the volume of the sphere:
V = \(\frac{1}{3}\) · r · B₁ + \(\frac{1}{3}\) · r · B₂ + \(\frac{1}{3}\) · r · B₃ + ………..
Factor out \(\frac{1}{3}\)r:
= \(\frac{1}{3}\)r(B₁ + B₂ + B₃)
The sum of the pyramids bases form the surface area of the sphere:
= \(\frac{1}{3}\)r(4πr²)
Use the commutative property of multiplication:
= \(\frac{4}{3}\) πr³