Practice the problems of Math in Focus Grade 7 Workbook Answer Key Chapter 4 Lesson 4.5 Real-World Problems: Algebraic Inequalities to score better marks in the exam.
Math in Focus Grade 7 Course 2 A Chapter 4 Lesson 4.5 Answer Key Real-World Problems: Algebraic Inequalities
Math in Focus Grade 7 Chapter 4 Lesson 4.5 Guided Practice Answer Key
Solve.
Question 1.
The average of 87, 90, 89, and a fourth number is at least 90. Describe the value of the fourth number.
Answer:
x = 94
Explanation:
The average of the 4 number is 90.
The sum of the numbers = 4 × 90
= 360
Let us consider the fourth number be ‘x’
The sum of the numbers = 360
87 +90 + 89 + x = 360
266 + x = 360
x = 360 – 266
x = 94
Copy and complete.
Question 2.
Grace is at the bookstore with $75 to spend. She plans to buy a reference book that costs $18 and some novels that cost $12 each. Find how many novels Grace can buy along with the reference book.
Let x be the number of novels Grace can buy. Define the variable.
+ 12x ≤ Write an inequality.
+ 12x – ≤ – Subtract from both sides.
12x ≤ Simplify.
12 ÷ ≤ ÷ Divide both sides by .
x ≤ Simplify.
Grace can buy at most novels.
Answer:
x ≤ 4.75
Explanation:
The equation is 12x+18 ≤ 75
Subtract 18 on both sides.
12x+18 – 18 ≤ 75 – 18
12x ≤ 57
Divide 12 on both sides.
12x ÷ 12 ≤ 57 ÷ 12
x ≤ 4.75
Question 3.
While Cheryl is on vacation, she wants to put her dog Cocoa in a kennel that offers an obedience class. She calls two boarding kennels to find their fees for their services.
After how many days will Best Dog Kennel be the cheaper option?
Let x be the number of days.
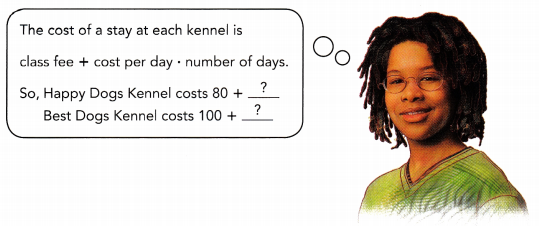
For Best Dog Kennel to be the cheaper option,
80 + > 100 + Write an inequality.
80 + – > 100 + – Subtract from both sides.
80 + > 100 Simplify.
80 + – 80 > 100 – 80 Subtract 80 from both sides.
> 20 Simplify.
÷ > 20 ÷ Divide both sides by .
x > Simplify.
Anytime after days, Best Dog Kennel will be the cheaper option.
Answer:
x > 4
Anytime after 4 days, Best Dog Kennel will be the cheaper option.
Explanation:
Happy dogs kernel costs 80 + 20x
Best dogs kernel costs 100 + 15x
80 + 20x > 100 + 15x
Subtract 15x on both sides.
80 + 20x – 15x > 100 + 15x – 15x
80 + 5x > 100
Subtract 80 on both sides.
80 + 5x – 80 > 100 – 80
5x > 20
Divide 5 on both sides.
5x ÷ 5 > 20 ÷ 5
x > 4
Anytime after 4 days, Best Dog Kennel will be the cheaper option.
Math in Focus Course 2A Practice 4.5 Answer Key
Solve. Show your work.
Question 1.
The perimeter of an equilateral triangle is at most 45 centimeters. Find the possible length of each side.
Answer:
The length of each side, a = 15
Explanation:
Perimeter of an equilateral triangle = 45 cms
Perimeter = 3a = 45cms
3a = 45
Divide 3 on both sides.
3a ÷ 3 = 45 ÷ 3
a = 15
Question 2.
Roger scored 1,800 points in four rounds of a debate competition. His opponent, Sawyer, scored 324 points in the first round, 530 points in the second round, and 619 points in the third round. How many points must Sawyer score in the final round to surpass Roger’s score?
Answer:
Sawyer should score more than 327 in the fourth round to surpass Roger’s score.
Explanation:
Roger scored 1800 points in four rounds.
Sawyer scored 324 + 530 + 619 + x in four rounds
‘x’ be the points sawyer scored in the fourth round.
Sawyer score in the final round to surpass Roger’s score:
324 + 530 + 619 + x > 1800
1473 + x > 1800
Subtract 1473 on both sides.
1473 + x – 1473 > 1800 – 1473
x > 327
Sawyer should score more than 327 in the fourth round.
Question 3.
Ben plans to sign up for a language class that will cost at least $195. His father gives him $75 and he earns $28 from mowing the lawn for his neighbors. Write and solve an inequality to find out how much more money he needs to save before he can sign up for the class.
Answer:
x + 75 + 28 ≥ 195; Ben needs to save, x ≥ 92
Explanation:
The equation for the above problem is, x + 75 + 28 ≥ 195
x + 103 ≥ 195
Subtract 103 on both sides.
x + 103 -103 ≥ 195 -103
x ≥ 92
Question 4.
In her last basketball game, Casey scored 46 points. In the current game, she has scored 24 points so far. How many more two-point baskets must she make if she wants her total score in her current game to be at least as great as her score in the last game?
Answer:
11 two-point baskets Casey should make to want her total score in the current game to be at least as great as the score in the last game.
Explanation:
Casey scored in the last basket ball game = 46
Casey scored in the current basket ball game = 24
Number of baskets she must make be ‘x’.
Let the equation be 24 + 2x ≥ 46
Subtract 24 on both sides.
24 + 2x – 24 ≥ 46 – 24
2x ≥ 22
Divide 2 on both sides.
2x ÷ 2 ≥ 22 ÷ 2
x ≥ 11
Question 5.
At Middleton Middle School, Marianne must score an average of at least 80 points on 4 tests before she can apply for the scholarship. If she scored 79, 81, and 77 for the first three tests, what must she score on her last test?
Answer:
The score of Marianne in her last test, x = 83
Explanation:
The average Marianne scored in the 4 tests = 80
The three tests scores are 79, 81 and 77
Let us consider the fourth test be ‘x’.
The total score = the no of tests × average of Marianne scores
= 4 × 80
= 320
79 + 81 + 77 + x = 320
237 + x = 320
Subtract 237 on both sides.
237 + x – 237 = 320 – 237
x = 83
Question 6.
At the movies, a bag of popcorn costs $3.50 and a bottle of mineral water costs $2.75. If Madeline has $18 and bought only 2 bottles of water, how many bags of popcorn can she buy at most?
Answer:
3.5 > x, Madeline can buy more than 3 bags.
Explanation:
Let the number of popcorn bags be x’
Given, 18 > 2 × 2.75 + 3.50x
18 > 5.5 +3.50x
Subtract 5.5 on both sides.
18 – 5.5 > 5.5 +3.50x – 5.5
12.5 > 3.50x
Divide 3.50 on both sides.
12.5 ÷ 3.50 > 3.50x ÷ 3.50
3.5 > x
Question 7.
Party favors are on sale for $2.40 each. You have $380 to spend on the decorations and gifts, and you have already spent $270 on decorations. Write and solve an inequality to find the number of party favors you can buy.
Answer:
approximately, we can buy 45 party favors.
Explanation:
Money you have to spend on decorations and gifts = 380
Money already spent = 270
Remaining money = Money you have to spend on decorations and gifts – Money already spent
= 380 – 270
=110
Party favors are on sale for $2.40 each.
Number of party favor = 2.40x
‘x’ be the number of party favors.
2.40x < 110
Divide 2.40 on both sides.
2.40x ÷ 2.40 < 110 ÷ 2.40
x < 45.8
So approximately, we can buy 45 party favors.
Question 8.
Charlie wants to join a golf club. He finds two clubs that have fees as shown in the table.
After how many months will Golf Club B be less expensive than Golf Club A?
Answer:
2 months.
Explanation:
Let us consider the number of months be ‘x’
80 + 45x > 110 + 30x
Subtract 30x on both sides.
80 + 45x – 30x > 110 + 30x – 30x
80 + 15x > 110
Subtract 80 on both sides.
80 + 15x – 80 > 110 – 80
15x > 30
Divide 15 on both sides.
15x ÷ 15 > 30 ÷ 15
x > 2
Question 9.
Molly can either take her lunch or buy it at school, It costs $1 .95 to buy lunch. If she wants to spend no more than $30 each month, how many lunches can she buy at most?
Answer:
15 days
Explanation:
Let us consider the number of days ‘x’
1.95x ≤ 30
Divide 1.95 on both sides.
1.95x ÷ 1.95 ≤ 30 ÷ 1.95
x ≤ 15.38
Approximately, 15 days
Question 10.
Tyson always likes to have at least $150 in his savings account. Currently he has $800 in the account. If he withdraws $35 each week, after how many weeks will the amount n his savings account be less than $150?
Answer:
18.5 weeks
Explanation:
Let us consider the number if weeks be ‘x’.
800 + 35x < 150
Subtract 150 on both sides.
800 + 35x – 150 < 150 – 150
35x < – 650
Divide 35 on both sides.
35x ÷ 35 < – 650 ÷ 35
x > 18.5
Question 11.
A cab company charges $0.80 per mile plus $2 for tolls. Melissa has at most $16 to spend on her cab fare. Write and solve an inequality for the maximum distance she can travel if she has at most $16 for cab fare. Can she afford to take a cab from her home to an airport that is 25 miles away?
Answer:
The maximum distance Melissa can travel is 17.5 miles. she cannot afford to travel to airport as 25 miles id greater than 17.5
Explanation:
Given, A cab company charges $0.80 per mile plus $2 for tolls.
Melissa has at most $16 to spend on her cab fare.
Let us consider the miles travelled e ‘x’.
0.8x + 2 ≤ 16
Subtract 2 on both sides.
0.8x + 2 – 2 ≤ 16 – 2
0.8x ≤ 14
Divide 0.8 on both sides.
0.8x ÷ 0.8 ≤ 14 ÷ 0.8
x ≤ 17.5
Question 12.
Nine subtracted from four times a number is less than or equal to fifteen. Write an inequality and solve it.
Answer:
4x-9≤15; x ≤ 6
Explanation:
Give, Nine subtracted from four times a number is less than or equal to fifteen
i.e; 4x-9≤15
Add 9 on both sides.
4x-9 + 9 ≤ 15 + 9
4x ≤ 24
Divide 4 on both sides.
4x ÷ 4 ≤ 24 ÷ 4
x ≤ 6
Question 13.
Sixteen plus five times a number is more than the number minus eight. Write an inequality and solve it.
Answer:
16 + 5x > x – 8 ; x > -6
Explanation:
Given, Sixteen plus five times a number is more than the number minus eight.
i.e; 16 + 5x > x – 8
Add 8 on both sides.
16 + 5x + 8 > x – 8 + 8
24 + 5x > x
Subtract x on both sides.
24 + 5x – x > x -x
24 +4x > 0
4x > -24
Divide 4 on both sides.
4x ÷ 4 > -24 ÷ 4
x > -6
Question 14.
Math Journal Write a word problem that can be solved using an inequality. Write the inequality that represents your problem. Then solve it.
Answer:
x = 2
Explanation:
Problem: four less than three times a number is equal to the same number.
3x – 4 = x
Subtract x on both sides.
3x – 4 – x = x – x
2x -4 = 0
Add 4 on both sides.
2x -4 + 4 = 0 + 4
2x = 4
Divide 2 on both sides.
2x ÷ 2 = 4 ÷ 2
x = 2
Brain @ Work
A father said, “My son is five times as old as my daughter. My wife is five times as old as my son and I am twice as old as my wife. Grandmother here, who is as old as all of us put together, is celebrating her 81st birthday today.” What ¡s the age of the man’s son?
Answer:
The age of man’s son is 5 years old.
Explanation:
Let us consider daughter’s age be ‘x’.
So, the son’s age is 5x
The wife’s age is “5 times old as son”, so 5 × 5x = 25x
His age is twice of this wife’s age, 2 × 25x = 50x
The sum of all the ages = 81
x + 5x + 25x + 50x = 81
81x = 81
Divide 81 on both sides.
81x ÷ 81 = 81 ÷ 81
x = 1
The age of man’s son is 5 years old.