Go through the Math in Focus Grade 5 Workbook Answer Key Chapter 1 Whole Numbers to finish your assignments.
Math in Focus Grade 5 Chapter 1 Answer Key Whole Numbers
Math Journal
Question 1.
Kim and Dominic found the sum of 8,642 and 9,328.

One of their answers is incorrect.
Show how you could use estimation to check which answer is reasonable.
Answer: Dominic’s answer is incorrect, Kim’s answer is correct.
Explanation:
If we add the given two numbers:8,642+9,328=17,970.
For this, we need to check estimation.
The numbers are 8,642 and 9,328
1. First, Find out the thousand’s digit in the number.
2. Next, choose the next smallest number (that is the hundredth digit of the number).
3. Now, check the hundred’s digit is either <5 (That means 0, 1, 2, 3, 4) or > = 5 (That is 5, 6, 7, 8, 9).
(i) If the digit is < 5, then the hundreds place is replaced with the digit ‘0’.
(ii) If the digit is > = 5, then the hundred’s digit is replaced with the digit ‘0’, and the thousand’s place digit is increased by 1 digit.
Step 1: Thousand’s digit of the number is 8 and 9.
Step 2: Hundred’s digit of the number is 6 and 3.
Step 3: The hundred’s digit ‘3’ is <5, then we have to apply 3(i) conditions. That is, the hundred’s placed is replaced with the digit ‘0’.
Step 4: The hundred’s digit ‘6’ is >=5, then we have to apply 3(ii) conditions. That is, the hundred’s placed is replaced with the digit ‘0’, and the thousand’s place digit is increased by 1 digit.
8,642 rounding of the nearest 1000 is equal to 9000.
9,328 rounding of the nearest 1000 is equal to 9000.
If we add both estimations then we get:
9000+9000=18000, which is near to Kim’s answer.
This is the process to estimate the value.
Question 2.
Samantha found these quotients.
a. 7,986 ÷ 8 = 998 R 2
Answer: 1000
998 is near to 1000 and this is the estimation process.
Number 7,986 Round to the Nearest 1000:
Step 1: Thousand’s digit of the number is 7.
Step 2: Hundred’s digit of the number is 9.
Step 3: The hundred’s digit ‘9’ is >=5, then we have to apply some conditions. That is, the hundred’s placed is replaced with the digit ‘0’ and the thousand’s place digit is increased by 1 digit.
7,986 rounding of the nearest 1000 is equal to 8000.
The estimation is 8000
Now divide with the given number that is 8:
8000÷8=1000.
b. 2,659 ÷ 3 = 264 R 3
Answer:1000
If we divide 2,659 with 3 then the quotient is 886.3333…
Number 2,659 Round to the Nearest 1000:
Step 1: Thousand’s digit of the number is 2.
Step 2: Hundred’s digit of the number is 6.
Step 3: The hundred’s digit ‘6’ is >=5, then we have to apply some conditions. That is, the hundred’s placed is replaced with the digit ‘0’ and the thousand’s place digit is increased by 1 digit.
2,659 rounding of the nearest 1000 is equal to 3000.
The estimation is 3000
Now divide with the given number that is 3:
3000÷3=1000.
Show how you could check whether the quotients are reasonable.
State in each case whether the quotient is reasonable.
Answer:
The quotients of the given numbers are 998.25 and 886.33
Step 1: For the first number that is (a) sum: 7,986 ÷ 8 = 998.
– Divide the given number by 8 then we will get the quotient of 998.25.
Step 2: For the second number that is (b) sum: 2,659 ÷ 3 = 264
– Divide the given number by 3 then we will get the quotient of 886.333.
If we take step 1 then the quotient is reasonable according to the given division and the estimation is also checked in the above sum(a). The estimation is 1000 which is near to 998.
If we take step 2 then the given quotient is wrong and the correct quotient for the sum (b) is 886.33. The estimation is also checked in the above sum (b). For this also the estimation is 1000 which is near to 886.
Question 3.
Liso was asked to round
a. 763 to the nearest hundred.
Answer:800
Explanation:
Number 763 Round to the Nearest 100:
Step 1: Hundred’s digit of the number is 7.
Step 2: Ten’s digit of the number is 6.
Step 3: The hundred’s digit ‘6’ is >=5, then we have to apply some conditions. That is, the ten’s placed is replaced with the digit ‘0’ and the hundred’s place digit is increased by 1 digit.
763 rounding of the nearest 100 is equal to 800.
b. 3,730 to the nearest thousand.
Answer: 4000
Explanation:
The number is 3730
Step 1: Thousand’s digit of the number is 3.
Step 2: Hundred’s digit of the number is 7.
Step 3: The hundred’s digit ‘7’ is >=5, then we have to apply conditions. That is, the hundred’s placed is replaced with the digit ‘0’, and the thousand’s place digit is increased by 1 digit.
3,730 rounding of the nearest 1000 is equal to 4000.
Lisa rounded 763 to 700 and 3,730 to 3,000. What mistakes did she make?
What should the correct answer in each case have been?
Answer: Both answers are wrong.
Explanation:
The first number Lisa rounded for 763=700
The second number Lisa rounded for 3,730=3000
The mistake she had done was rounding down the values that are 700 and 3000.
There are some conditions to round up and down the values. The conditions are:
1. First, Find out the thousand’s digit in the number.
2. Next, choose the next smallest number (that is the hundredth digit of the number).
3. Now, check the hundred’s digit is either <5 (That means 0, 1, 2, 3, 4) or > = 5 (That is 5, 6, 7, 8, 9).
(i) If the digit is < 5, then the hundreds place is replaced with the digit ‘0’.
(ii) If the digit is > = 5, then the hundred’s digit is replaced with the digit ‘0’, and the thousand’s place digit is increased by 1 digit.
According to the above conditions we need to round the nearest values.
The correct answers for 763 and 3,730 are 800 and 4000.
put on your thinking cap!
Challenging Practice
Arrange the digits to form three 6-digit numbers that will round to 756,000 when rounded to the nearest thousand.

Answer:
The number is 756,000
Number 756,000 Round to the Nearest 1000:
Step 1: Thousand’s digit of the number is 6.
Step 2: Hundred’s digit of the number is 0.
Step 3: The hundred’s digit ‘0’ is <5, then we have to apply some conditions. That is, the hundred’s placed is replaced with the digit ‘0’
756,000 rounding of the nearest 1000 is equal to 756,000.
The three 6-digit numbers are 755,678, 756,052, 756,275.
If we calculate these 3 numbers these all are round to 756,000 when rounded to the nearest thousand.
Take number 755,678:
Step 1: Thousand’s digit of the number is 5.
Step 2: Hundred’s digit of the number is 6.
Step 3: The hundred’s digit ‘6’ is >=5, then we have to apply some conditions. That is, the hundred’s placed is replaced with the digit ‘0’, and the thousand’s place digit is increased by 1 digit.
So the number is 756,000. The hundred thousand and ten thousand places will not change because in the question asked round the number nearest to thousand.
According to the above conditions we need to round the nearest values. Likewise, write the other two numbers also.
put on your Thinking cap
Problem Solving
Question 1.
What number can you subtract from 3,200 so that their difference is a 4-digit number that has: the digit 2 in the thousands place, the digit 3 in the hundreds place and zeros in the tens and ones place?
Answer: 900
Explanation:
The given number=3,200
The number we need to subtract to get the number 2,300=X
The number need to come if we subtract X and 3,200=2,300.
If we take 2,300: The value of digits are 0-ones place, 0-tens place, 3-hundreds place, 2-thousands place.
So, If we subtract 3,200 from 900 then we get the 2,300 because we need to take borrow from another number.
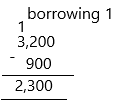
Here I take borrow 1 from 3 because we cannot subtract 9 from 2. If we take borrow from another number then it will become 12, from 12 we can easily subtract 9. Then it becomes 3. Finally, if we subtract 900 from 3,200 then we get 2,300.
Therefore, the value of X is 900.
Another method:
By math sentence, we can write as:
3200-X=2300
Taking X to the right side then it becomes:
3200=2300+x
If we take all the numbers into one side then it becomes:
3200-2300=X
900=X
Therefore, X=900.
Question 2.
A 3-digit number when divided by 5 has an even quotient. When it is divided by 3, it also has an even quotient.
a. What is the digit in the one’s place?
Answer: 0
Explanation:
It has to be a multiple of 5. All multiples of 5 either end in 0 or 5. Since it has to be even it has to end in 0, since if it ended in 5 it would be odd. Therefore, the answer to this must be 0, because the digit on the right end of the number is one’s digit.
b. What can the number be?
Answer:
Since it ends with a zero it is divisible by 10. So if you divide it by 10, the quotient will be a two-digit number which is the same as the number without the 0 on the right end.
But the quotient will still be divisible by 3.
So the number could be any two-digit number that is multiple of 3 with a zero on the right end of it for its one’s digit.
The smallest two-digit number that is multiple of 3 is 12. So the smallest number could be is 120.
The largest two-digit number that is a multiple of 3 is 99. So the largest number could be is 990.
So it could be any of these thirty 3-digit numbers:
120, 150, 180, 210, 240,270, 300, 330, 360, 390, 420, 450, 480, 510, 540, 570, 600, 630, 660, 690, 720, 750, 780, 810, 840, 870, 900, 930, 960, 990.